2D Physics Engine Tutorial – Part 2
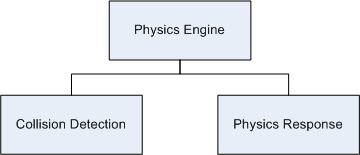
In part 1 I explained what a physics engine does and what kind of physics engines there exists. This post will focus on the collision detection internals of physics engines. Overview A physics engine does two things: Collision detection and physics reaction. They work seamless together to create the illusion of things colliding and bouncing back. Collision Detection The collision detection part of the engine is responsible for detecting when things overlap and by how much they overlap. Most physics engines have developed a two-tier architecture where you first detect if objects are near each other using a crude representation of the object. Then it performs the collision detection on the objects itself using its real representation. It basically is setup like this: Broad phase The broad phase is the one responsible for detecting objects near each other. It was developed to speed up the collision detection phase by reducing the amount of collision checks needed. Imag